Exact Definition of Dirac Operator
Let $(M,g)$ be an orientable pseudo-riemannian manifold. Each tangent space $T_xM$ is a pseudo-euclidean space and hence has an associated Clifford algebra $CL(T_xM)$, which is the fibre at $x\in M$ of the Clifford bundle $Cl(TM)$. If the manifold is spin (a topological condition which says that the oriented orthonormal frame bundle lifts to a spin bundle) then it admits a vector bundle $\Sigma$ whose fibre at $x$ is an irreducible Clifford module of $Cl(T_xM)$. This means that we have a bundle map $$Cl(TM) \times \Sigma \to \Sigma$$ given fibrewise by the Clifford action of $Cl(T_xM)$ on $\Sigma_x$.
(Please allow me the notational abuse of confusing bundles with their sections, to save me some writing.)
Because we have a metric, we have musical isomorphisms $$\flat: TM \to T^*M \qquad\text{and}\qquad \sharp: T^*M \to TM~,$$ the latter of which can be composed with the above Clifford action to arrive at $$Cl(T^*M) \times \Sigma \xrightarrow{\operatorname{cl}} \Sigma~.$$
The Spin group $Spin(T_xM)$ is contained in $Cl(T_xM)$ and hence acts on $\Sigma_x$ by restriction. This representation need not be irreducible; although if $M$ is odd-dimensional it is. This allows us to view $\Sigma$ as a bundle of spinors, associated to a (not necessarily irreducible) spinor representation of the spin group. In fact, it is an associated vector bundle of the spin bundle.
The Levi-Civita connection on $TM$ defines an Ehresmann connection on the bundle of oriented orthonormal frames. That connection lifts to a connection on the spin bundle and hence induces a Koszul connection on $\Sigma$, called the spin connection. We thus get a bundle map $$ \Sigma \xrightarrow{\nabla} T^*M \otimes \Sigma $$
The Dirac operator is now simply the composition $$ \Sigma \xrightarrow{\nabla} T^*M \otimes \Sigma \xrightarrow{\operatorname{cl}} \Sigma~.$$
Thus the domain and range are the sections of $\Sigma$, the so-called spinor fields.
If $M$ is even-dimensional (and depending on signature perhaps after complexifying) then one can refine this. Under spin, $\Sigma$ splits into eigenbundles of the Clifford action by the volume form: $$ \Sigma = \Sigma_+ \oplus \Sigma_-$$ Then $\nabla : \Sigma_\pm \to \Sigma_\pm$, but $$ \operatorname{cl}: T^*M \otimes \Sigma_\pm \to \Sigma_\mp$$ whence now the Dirac operator, defined in the same way, breaks up into two maps $\Sigma_\pm \to \Sigma_\mp$.
Added
I'm adding more details to answer Igor's question. If we are in signature $(s,t)$, with $n=s+t$, being the dimension, then the answer depends on $s-t \mod 8$:
- $s-t = 0 \mod 8$: $\Sigma$ is real and $\Sigma = \Sigma_+ \oplus \Sigma_-$, where $\Sigma_\pm$ are real.
- $s-t = 1 \mod 8$: $\Sigma$ is complex but has a spin-invariant real structure: it does not split.
- $s-t = 2 \mod 8$: $\Sigma$ is quaternionic and it splits if we complexify: $\Sigma_{\mathbb{C}} = \Sigma_+ \otimes \Sigma_-$, with $\Sigma_\pm$ complex conjugates.
- $s-t = 3 \mod 8$: $\Sigma$ is quaternionic and remains so under spin: it does not split.
- $s-t = 4 \mod 8$: $\Sigma$ is quaternionic and it splits $\Sigma = \Sigma_+ \oplus \Sigma_-$, where $\Sigma_\pm$ are also quaternionic.
- $s-t = 5 \mod 8$: $\Sigma$ is complex but has a spin-invariant quaternionic structure: it does not split.
- $s-t = 6 \mod 8$: $\Sigma$ is real and if we complexify then it splits $\Sigma_{\mathbb{C}} = \Sigma_+ \otimes \Sigma_-$, with $\Sigma_\pm$ complex conjugates.
- $s-t = 7 \mod 8$: $\Sigma$ is real, does not split and remains real.
I would think that these these notes by Akhil Mathew provide the "exact definition" you are asking for:
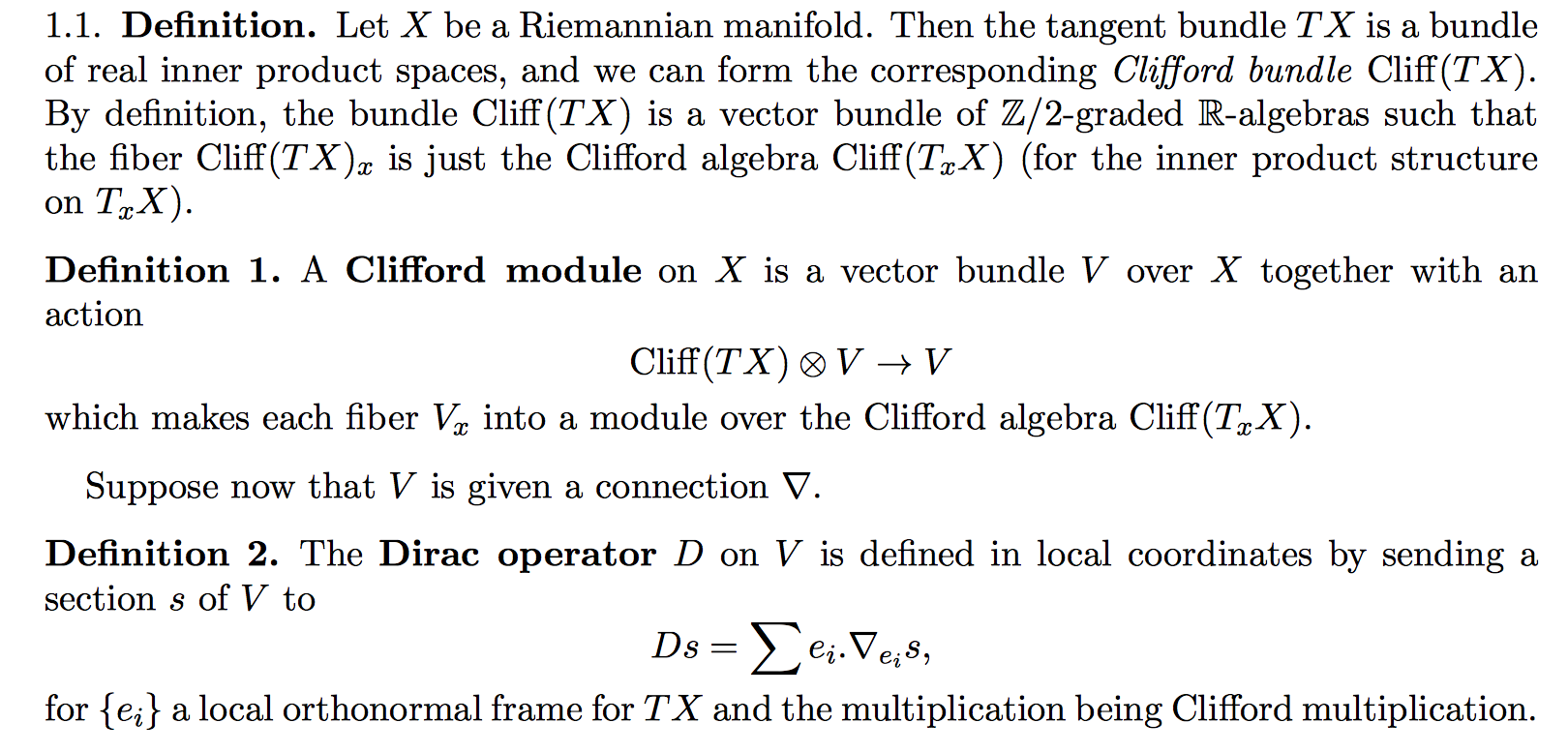
In response to the follow-up question "which is the first Clifford module used in the physics context":
Two different representations (modules) of the Clifford algebra were studied in early work on the Dirac equation. Paul Dirac himself used a quaternionic representation, while Ettore Majorana used a real representation.
The physics implications were entirely different: Dirac had a complex field equation, and concluded that the field and its complex conjugate described two different particles. One was the electron, the complex conjugate was unknown at the time. Dirac hypothesised that it described a positively charged "antiparticle", with the same mass as the electron. This "positron" was discovered shortly afterwards, a triumph of mathematical physics.
Majorana, in contrast, had a real wave equation and hypothesised that it would describe charge-neutral particles that were their own antiparticle. We still do not know whether such particles exist in nature (the neutrino may or may not be of this type).
Historical note: the real representation of the Dirac equation is called the Majorana equation, but this was actually a rediscovery: Eddington had published it a decade before Majorana, so "Eddington-Majorana equation" would be a more appropriate name.